

Ouyang, ``Permutation-invariant quantum codes,'' Phys. van Loock, ``Approximate quantum error correction for generalized amplitude-damping errors,'' Phys. Shi, ``Sparse Quantum Codes from Quantum Circuits,'' in Proceedings of the Forty-Seventh Annual ACM on Symposium on Theory of Computing, STOC '15 (ACM Press, New York, NY, USA, 2015) pp. Bravyi, ``Subsystem codes with spatially local generators,'' Phys. Delfosse, ``Tradeoffs for reliable quantum information storage in surface codes and color codes,'' in 2013 IEEE International Symposium on Information Theory (Institute of Electrical & Electronics Engineers (IEEE), 2013) arXiv:1301.6588. Terhal, ``A no-go theorem for a two-dimensional self-correcting quantum memory based on stabilizer codes,'' New Journal of Physics 11, 043029 (2009), arXiv:0810.1983. Kastoryano, ``Entanglement renormalization, quantum error correction, and bulk causality,'' (2017), arXiv:1701.00050. Terhal, ``Noise thresholds for the -concatenated toric code,'' Quant. Doherty, ``Toric codes and quantum doubles from two-body Hamiltonians,'' New Journal of Physics 13, 053039 (2011), arXiv:1011.1942. Preskill, ``Logical operator tradeoff for local quantum codes,'' Phys. Terhal, ``Tradeoffs for reliable quantum information storage in 2D systems,'' Phys. Michalakis, ``Topological quantum order: stability under local perturbations,'' Journal of Mathematical Physics 51, 093512 (2010a), arXiv:1001.0344. Wen, ``Quasiadiabatic continuation of quantum states: The stability of topological ground-state degeneracy and emergent gauge invariance,'' Physical Review B 72, 045141 (2005), cond-mat/0503554. Zwolak, ``Stability of frustration-free Hamiltonians,'' Communications in Mathematical Physics 322, 277–302 (2013), arXiv:1109.1588. Kitaev, ``Anyons in an exactly solved model and beyond,'' Annals of Physics 321, 2–111 (2006), cond-mat/0506438. Read, ``Nonabelions in the fractional quantum hall effect,'' Nuclear Physics B 360, 362–396 (1991). Cramer (Springer Berlin Heidelberg, Berlin, Heidelberg, 2005) pp. Smith, ``Approximate quantum error-correcting codes and secret sharing schemes,'' in Advances in Cryptology – EUROCRYPT 2005: 24th Annual International Conference on the Theory and Applications of Cryptographic Techniques, Aarhus, Denmark, May 22-26, 2005.
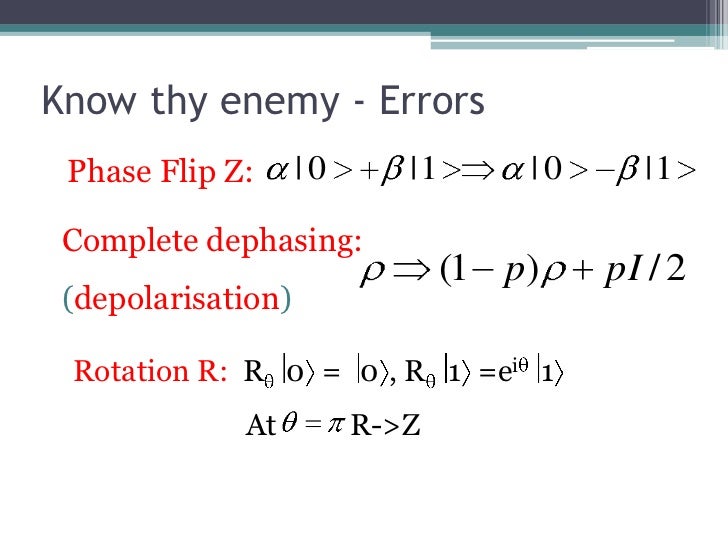
Yamamoto, ``Approximate quantum error correction can lead to better codes,'' Phys. (American Mathematical Society, 2010) pp.

Gottesman, ``An introduction to quantum error correction and fault-tolerant quantum computation,'' in Quantum Information Science and Its Contributions to Mathematics, Vol. Terhal, ``Quantum error correction for quantum memories,'' Rev. This supports one of the assumptions of algebraic anyon theories, that there exist only finitely many anyon types.
#Steinspring quantum error correction code#
Finally, we prove that for two-dimensional systems if logical operators can be approximated by operators supported on constant-width flexible strings, then the dimension of the code space must be bounded.

In a regime where the recovery is successful to accuracy $\delta$ that is exponentially small in $\ell$, which is the case for perturbations of local commuting projector codes, our bound reads $kd^\bigr)$ in operator norm. Our tradeoff bounds relate the number of physical qubits $n$, the number of encoded qubits $k$, the code distance $d$, the accuracy parameter $\delta$ that quantifies how well the erasure channel can be reversed, and the locality parameter $\ell$ that specifies the length scale at which the recovery operation can be done. We introduce a notion of local approximate correctability and code distance, and give a number of equivalent formulations thereof, generalizing various exact error-correction criteria. We study the fundamental limits on the reliable storage of quantum information in lattices of qubits by deriving tradeoff bounds for approximate quantum error correcting codes.


 0 kommentar(er)
0 kommentar(er)
